Spatial Pattern Formation

Spatial patterns are widely present in different natural dynamical systems. Their occurrence has been studied for quite a long time with several applications in different fields, from hydrodynamic systems (e.g. Rayleigh-Bénard convection), plant ecosystems (e.g. dryland and riparian vegetation), to biochemical and neural systems. The study of patterns can offer useful information on the underlying processes causing possible changes of the system. Deterministic mechanisms in pattern formation have been widely studied, while stochastic models have only been developed more recently. They explain pattern formation as a noise-induced effect in the sense that patterns can emerge as a consequence of the randomness of the system's fluctuations.

The spatio-temporal dynamics is expressed in terms of stochastic PDE describing the formation of ordered anc coherent structures as a consequence of fluctuations and disturbances of the system. We propose an overview of the main stochastic processes related to the presence of a Gaussian white noise. In particular, we focus on the main components playing a crucial role in noise-induced pattern formation:

  • a deterministic local dynamics, which tends to drive the field variable to a uniform steady state;
  • a (multiplicative or additive) noise component, able to maintain the dynamics away from the uniform steady state;
  • a diffusive spatial coupling term, which provides spatial coherence.

The spatial coupling can be simply diffusive (Laplacian) or can represent the Swift-Hohemberg mechanism. Both couplings are relevant within the pollutant dispersion dynamics and in fluid mechanics. In particular, the Swift-Hohemberg coupling derives from the Rayleigh-Bénard convective cells. The noise component can be periodic and/or stochastic. The presence of a temporal periodicity leads to oscillating patterns which periodically emerge and disappear (stochastic resonance). The stochatic term, which can be modelled as Gaussian, shot or dichotomic noise, well fits different kinds of high-order forcings, such as the presence of turbulent forcings.

We call patterned a field that exhibits an ordered state with organized spatial structures. This general definition, including both periodic as well as multiscale patterns, is often adopted in the environmental sciences, where the number of different processes can prevent the organization of the system with a clear dominant wavelength. Since noisy fluctuations are always present in real systems and pattern formation, here described, is comletely noise-induced, randomness can actually promote spatial coherence in different environmental processes.

 

Figure 1: Additive Noise (Steady and Multiscale Patterns). Numerical simulation of the spatial field Φ at different instants, t=0, 10, 100. See the MOVIE.

 

Figure 2: Additive Noise: Comparison with Vegetation Patterns. (left) Aerial photograph of vegetation pattern in New Mexico (34°47'N, 108°21'O) and (right) numerical simulation at t=100. Google Earth imagery © Google Inc. Used with permission.

 

Figure 3: Multiplicative Noise (Steady and Periodic Patterns). Numerical simulation of the spatial field Φ at different instants, t=0, 10, 100. See the MOVIE.

 

Figure 4: Multiplicative Noise (Transient and Multiscale Patterns). Numerical simulation of the spatial field Φ at different instants, t=0, 10, 40. See the MOVIE.

 

Figure 5: Stochastic Resonance (Time oscillating and Multiscale Patterns). Numerical simulation of the spatial field Φ at different instants, t=500, 590, 682.5. See the MOVIE.

 

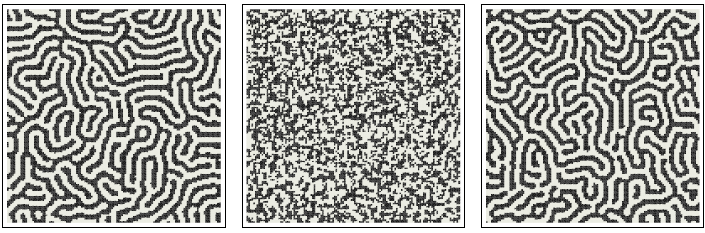
Figure 6: Stochastic Resonance (Time oscillating and Periodic Patterns). Numerical simulation of the spatial field Φ at different instants, t=110, 140, 195. See the MOVIE.

 

Related Publications